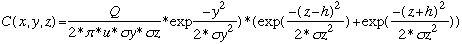
One of the key assumptions of this model is that over short periods of time (such as a few hours) steady state conditions exists with regard to air pollutant emissions and meteorological changes. Air pollution is represented by an idealized plume coming from the top of a stack of some height and diameter. One of the primary calculations is the effective stack height. As the gases are heated in the plant (from the burning of coal or other materials), the hot plume will be thrust upward some distance above the top of the stack -- the effective stack height. We need to be able to calculate this vertical displacement, which depends on the stack gas exit velocity and temperature, and the temperature of the surrounding air.
Once the plume has reached its effective stack height, dispersion will begin in three dimensions. Dispersion in the downwind direction is a function of the mean wind speed blowing across the plume. Dispersion in the cross-wind direction and in the vertical direction will be governed by the Gaussian plume equations of lateral dispersion. Lateral dispersion depends on a value known as the atmospheric condition, which is a measure of the relative stability of the surrounding air. The model assumes that dispersion in these two dimensions will take the form of a normal Gaussian curve, with the maximum concentration in the center of the plume.
The "standard" algorithm used in plume studies is the Gaussian plume model, develped in 1932 by O.G. Sutton. The algorithm is as follows:
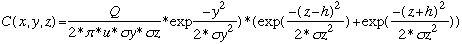
where:
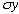and 
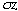are the standard deviations of a statistically normal plume in the lateral and vertical dimensions, respectively
This algorithm has been shown in a number of studies to be fairly predictive of emission dispersion in a variety of conditions. If you look at some of the examples on other Web links, you will find its application in roadside, urban, and long-term conditions. In this algorithm, we are concerned with dispersion in all three dimensions (x, y, and z):
The other major calculations for a simple Gaussian plume model are as follows:
The stability categories were developed in the late 1970s, and are based on wind speed, insolation, and extent of cloud cover. As shown above, we can calculate the values the standard deviations from the downwind axis for these six conditions or categories using the algorithms above.
Initially, Gaussian plume models were used for pollutants such as carbon monoxide and other non-reactive species. The model has serious limitations when trying to account for pollutants that undergo chemical transformation in the atmosphere. Coupled with its dependence on steady state meteorological conditions and its short-term nature, this model has substantial limitations for use as a long-term airshed pollutant evaluator.
An interactive Gaussian plume case study and model are available to you through the next few sections. Use these to explore the types of inputs and outputs common to a Gaussian Plume Model.
The Shodor
Education Foundation, Inc.
